площадь под графиком матлаб

Видео:MatLab. Урок 3. Функции и построение графиков.Скачать

Matlab, как рассчитать AUC (площадь под кривой)?

у меня есть файл data.txt С двумя столбцами и N строками, что-то вроде этого:

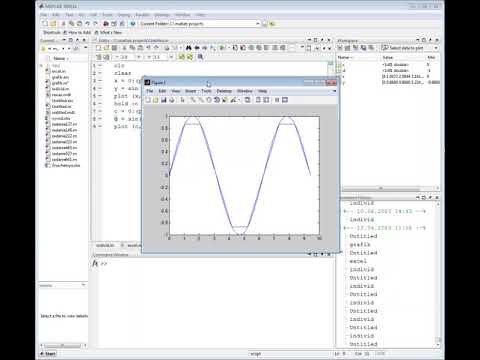
каждая пара значений в файле соответствует одной точке координат (x,y). При построении графика эти точки образуют кривую. Я хотел бы рассчитать площадь под кривой (AUC) этой кривой.

поэтому я загружаю данные:

и X содержит все X-координаты точек, а Y все координаты y.

как я мог вычислить площадь под кривой (AUC) ?

Видео:MATLAB 07 Интерактивное построение графиковСкачать

6 ответов

самый простой способ-трапециевидная функция правила trapz .

Если ваши данные, как известно, гладкие, вы можете попробовать использовать правило Симпсона, но нет ничего встроенного в MATLAB для интеграции числовых данных через правило Симпсона. (&Я не уверен, как использовать его для данных x/y, где x не увеличивается стабильно)

просто добавить AUC = trapz(X, Y) к вашей программе и вы получите площадь под кривой

Видео:Построение графиков: нахождение площади под кривойСкачать

Площадь под графиком матлаб

Filled area 2-D plot

Видео:MATLAB 08 Расширенное построение графиков: особенности и приемыСкачать

Syntax

Видео:MatLab. Создание 3D графиков. Практика 4Скачать

Description

area( X , Y ) plots the values in Y against the x-coordinates X . The function then fills the areas between the curves based on the shape of Y :

If Y is a vector, the plot contains one curve. area fills the area between the curve and the horizontal axis.

If Y is a matrix, the plot contains one curve for each column in Y . area fills the areas between the curves and stacks them, showing the relative contribution of each row element to the total height at each x-coordinate.

area( Y ) plots Y against an implicit set of x-coordinates and fills the areas between the curves.

If Y is a vector, the x-coordinates range from 1 to length(Y) .

If Y is a matrix, the x-coordinates range from 1 to the number of rows in Y .

area( ___ , basevalue ) specifies the baseline value for the area plot. basevalue corresponds to a horizontal baseline. area fills the area confined between the curves and this line. Specify basevalue as the last argument in any of the previous syntaxes.

area( ___ , Name,Value ) modifies the properties of the area plot using one or more name-value pair arguments. The properties apply to all of the displayed areas. For example, ‘LineStyle’,’—‘ specifies a dashed line style for the plot. Specify the name-value pairs after all of the arguments in any of the previous syntaxes. For a list of properties, see Area Properties .

area( ax , ___ ) displays the area plot in the target axes. Specify the axes as the first argument in any of the previous syntaxes.

a = area( ___ ) returns one or more Area objects. The number of objects is equal to the number of plotted areas. Use a to modify properties of the areas after creating them. For a list of properties, see Area Properties .

Видео:Математика это не ИсламСкачать

Examples

Create Area Plot with One Curve

Create a vector of four values. Display the values in an area plot.

Create Area Plot with Multiple Curves

Create matrix Y . Then display the values in Y as an area plot. Because Y contains three columns, area plots three curves and stacks them.

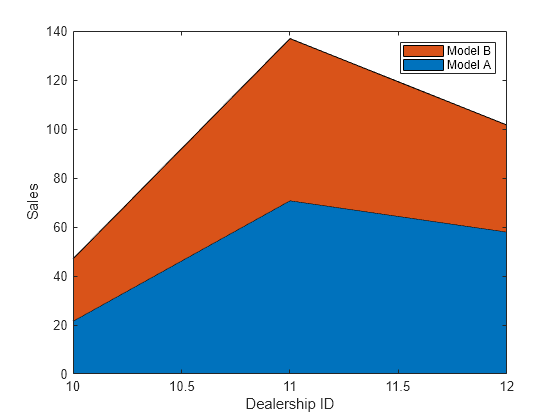
Create Area Plot Using Horizontal Axis Values

Define x as a vector of three car dealership IDs. Define Y as a matrix containing the number of cars sold per model. Display the values in the matrix in an area plot. Then add the axis labels and a legend.

Set the tick marks along the x -axis to correspond to the values in x .

Adjust Baseline Value of Area Plot

Create matrix Y . Then display the values of Y in an area plot with a baseline value of -4 . area fills the areas specified by the curves and the line y = -4 .

Specify Line Style of Area Plot

Create matrix Y . Display the values of Y in an area plot that uses a dotted line style.

Plot Filled Area into Target Axes

Create a tiled chart layout in the ‘flow’ tile arrangement, so that the axes fill the available space in the layout. Next, call the nexttile function to create an Axes object and return it as ax1 . Display an area plot by passing ax1 to the area function.

Repeat the process to create a second Axes object and a second area plot.

Change Area Characteristics After Plotting

Create matrix Y . Then create an area plot, specifying an output argument when calling the area function. In this case, area returns a vector of three Area objects. Each object corresponds to a different column of Y .

Modify the second area to be green with thick red edges.

Set Colors in Area Plot

Display an area plot with three curves.

Set the color order to blue, purple, and gray.

Видео:Как оформить график в MatLab.Скачать

Input Arguments

X — x-coordinates
vector | matrix

x-coordinates, specified as a vector or matrix. The size and shape of X depend on the shape of your data and the type of plot you want to create. This table describes the most common situations.

Specify X and Y as any combination of row or column vectors of the same length. For example:

area plots one filled area for each column of Y and stacks the areas. Specify Y as a matrix and X as a row or column vector with length equal to the number of rows in Y . For example:

You also can specify X as a matrix with the same size as Y . To avoid unexpected output when X is a matrix, specify X with identical columns.

Data Types: single | double | int8 | int16 | int32 | int64 | uint8 | uint16 | uint32 | uint64 | categorical | datetime | duration

Y — y-coordinates
vector | matrix

y-coordinates, specified as a vector or matrix. The size and shape of Y depend on the shape of your data and the type of plot you want to create. This table describes the possible situations.

Specify X and Y as any combination of row or column vectors of the same length. For example:

area plots one filled area for each column of Y and stacks the areas. Specify Y as a matrix and X as a row or column vector with length equal to the number of rows in Y . For example:

You also can specify X as a matrix with the same size as Y . To avoid unexpected output when X is a matrix, specify X with identical columns.

Data Types: single | double | int8 | int16 | int32 | int64 | uint8 | uint16 | uint32 | uint64 | duration

basevalue — Baseline value
0 (default) | numeric scalar

Baseline value, specified as a numeric scalar. The baseline value specifies the y-coordinate of a horizontal baseline. area fills the area confined between the data curves and this baseline.

ax — Target axes
Axes object

Target axes, specified as an Axes object. If you do not specify the axes, then area displays the plot in the current axes.

Name-Value Arguments

Specify optional comma-separated pairs of Name,Value arguments. Name is the argument name and Value is the corresponding value. Name must appear inside quotes. You can specify several name and value pair arguments in any order as Name1,Value1. NameN,ValueN .

Example: area([1 2 3],’FaceColor’,’r’) specifies a red fill color for the area.

Note

The properties listed here are only a subset. For a complete list, see Area Properties .

FaceColor — Area fill color
RGB triplet | hexadecimal color code | ‘r’ | ‘g’ | ‘b’ | ‘flat’ | .

Area fill color, specified as an RGB triplet, a hexadecimal color code, a color name, or ‘flat’ .

Starting in R2017b, the default value is an RGB triplet from the ColorOrder property of the axes. In previous releases, the default value was ‘flat’ and the colors were based on the colormap.

For a custom color, specify an RGB triplet or a hexadecimal color code.

An RGB triplet is a three-element row vector whose elements specify the intensities of the red, green, and blue components of the color. The intensities must be in the range [0,1] ; for example, [0.4 0.6 0.7] .

A hexadecimal color code is a character vector or a string scalar that starts with a hash symbol ( # ) followed by three or six hexadecimal digits, which can range from 0 to F . The values are not case sensitive. Thus, the color codes ‘#FF8800’ , ‘#ff8800’ , ‘#F80’ , and ‘#f80’ are equivalent.

Alternatively, you can specify some common colors by name. This table lists the named color options, the equivalent RGB triplets, and hexadecimal color codes.

Видео:Построение освещенной поверхности. Высокоуровневая графика. Урок 37Скачать

MATLAB — Интеграция

Интеграция имеет дело с двумя принципиально разными типами проблем.

В первом типе дается производная функции, и мы хотим найти функцию. Поэтому мы в основном обращаем вспять процесс дифференциации. Этот обратный процесс известен как антидифференцирование, или нахождение примитивной функции, или нахождение неопределенного интеграла .

Второй тип проблем включает сложение очень большого количества очень маленьких величин, а затем определение предела, когда размер величин приближается к нулю, а число членов стремится к бесконечности. Этот процесс приводит к определению определенного интеграла .

В первом типе дается производная функции, и мы хотим найти функцию. Поэтому мы в основном обращаем вспять процесс дифференциации. Этот обратный процесс известен как антидифференцирование, или нахождение примитивной функции, или нахождение неопределенного интеграла .

Второй тип проблем включает сложение очень большого количества очень маленьких величин, а затем определение предела, когда размер величин приближается к нулю, а число членов стремится к бесконечности. Этот процесс приводит к определению определенного интеграла .

Определенные интегралы используются для определения площади, объема, центра тяжести, момента инерции, работы, выполняемой силой, и во многих других приложениях.

Видео:Matlab. Создание 2D графиков. Практика 3 часть1Скачать

Поиск неопределенного интеграла с помощью MATLAB

По определению, если производная функции f (x) есть f ‘(x), то мы говорим, что неопределенный интеграл от f’ (x) по x равен f (x). Например, поскольку производная (по x) x 2 равна 2x, мы можем сказать, что неопределенный интеграл от 2x равен x 2 .

f ‘(x 2 ) = 2x , следовательно,

Неопределенный интеграл не является уникальным, потому что производная x 2 + c для любого значения константы c также будет 2x.

Это выражается в символах как —

Где c называется «произвольной константой».

MATLAB предоставляет команду int для вычисления интеграла выражения. Чтобы вывести выражение для неопределенного интеграла функции, мы пишем —

Например, из нашего предыдущего примера —

MATLAB выполняет приведенный выше оператор и возвращает следующий результат —

Пример 1

В этом примере давайте найдем интеграл некоторых часто используемых выражений. Создайте файл сценария и введите в нем следующий код —

Когда вы запускаете файл, он показывает следующий результат —

Пример 2

Создайте файл сценария и введите в нем следующий код —

Обратите внимание, что функция pretty возвращает выражение в более читаемом формате.

📽️ Видео

Высокоуровневая графика. Построение графиков из окна Workspace. Урок 24Скачать

Графики в MATLABСкачать

Matlab. Лекция 17. Высокоуровневая 3D графика.Скачать

Метод прямоугольников для нахождения определенного интегралаСкачать

Построение кривой в полярной системе координатСкачать

Как создавать функции в MATLABСкачать

Построение трехмерных графиков в MatlabСкачать

Matlab: взаимодействие с Excel, Simulink. Построение графиков в Matlab.Скачать

Построение графиков и диаграммСкачать

Диаграммы векторных данных 1. Высокоуровневая графика. Урок 25Скачать